En supposant le gain  connu, il est possible de calculer l'erreur d'analyse
commise en partant de l'Eq. 4.1 et en utilisant les erreurs introduites
auparavant :
connu, il est possible de calculer l'erreur d'analyse
commise en partant de l'Eq. 4.1 et en utilisant les erreurs introduites
auparavant :
Comme les erreurs d'ébauche et d'observation sont décorrélées, alors la
matrice de covariance d'erreur d'analyse est alors égale à:
En reprenant l'estimation de
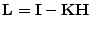 obtenue avec l'hypothèse d'une
erreur d'analyse sans biais, la matrice de covariance d'erreur d'analyse
s'écrit alors sous la forme :
obtenue avec l'hypothèse d'une
erreur d'analyse sans biais, la matrice de covariance d'erreur d'analyse
s'écrit alors sous la forme :
Nicolas Daget
2007-11-16